 |
 Knots, braids, bends, links, hitches, weaves etc. are described by d-dimensional space-curves. Their projections can be shown as 2D shadows, 2.5D knot diagrams, and 3D tubes using piece-wise cubic interpolation. Mathematical knots and links are closed 3D lines that cannot be untangled without passing lines through lines. Braids provide compact knot descriptors; bends join two ropes; hitches and binding knots are tied round posts etc.; many are illustrated.
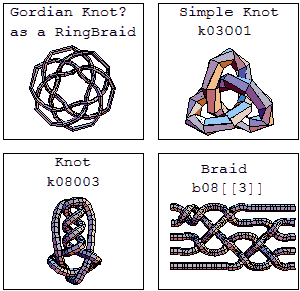
A small database is included; the much larger K2K database can be accessed. Elementary knot creation and basic knot-analysis procedures are supplied, together with a gallery of knot-graphs, a glossary and some key knot references (several on-line). Many aspects of knots are discussed, and the procedures are demonstrated, in a tutorial containing 44 examples. Typical nomenclature: k08003, b08003 and kg08003 are the knot, the braid, and the knot graph corresponding to the knot called 08-003 or 8(subscript 3) elsewhere, as the third simple knot with eight crossings in the standard list. A1431 is knot number 1431 in The Ashley Book of Knots. Adamsfig28 is from The Knot Book.
Local piece-wise cubic interpolation fits chords to smooth lines between sets of points with any number of coordinates. The slope at each point is that between the neighbouring points, and a tension option (with linear interpolation corresponding to infinite tension) controls the shape. The curve is closed smoothly (creating a loop) if the first and last points coincide Originally SpaceCurvKnots, April 2005. Updated by the author to KnotsEtc, June 17th, July 18th, July 24th, Aug. 24 2005.
|
|
For the newest resources, visit Wolfram Repositories and Archives »
